The distance to the stars
Look at the image of a starfield to the right. Can you tell which of the two stars, A or B, is closer?
If you selected A, on what did you base your choice?
1. Star A is much larger than B so should be closer.
OR
2. Star A is brighter than B so must be closer.
Place your mouse pointer over the image and observe what happens.
As you can see, Star B appears to move relative to the other stars. When you remove your mouse from the image it appears to move back to its original relative position.
Imagine that this to and fro motion took place over 12 months. What could account for this shift in position?
Parallax
If you said it is due to our orbit around the Sun you would be correct. This shift in a star's relative position is due to parallax. You can try this yourself by holding a finger about 20 cm from your face and look at its position relative to a more distant object. As you close your left eye, the finger seems to shift to the left. When you open that and close the right eye, the finger shifts to the right. What happens to this parallax shift if you move your finger out to arms length?
The parallax of stars was predicted by Copernicus' heliocentric model but was not observed with the naked-eye. Even Galileo was unable to detect any stellar motion when he used a telescope. He correctly inferred that this was due to the vast distance to stars. The first measurement of parallax was not made until 1838.
The reason that star B appears to move is that it is closer to us than the other stars, even though star A is brighter. As you can see then, the distance to a star cannot be determined by its brightness (or size on photographic plate or CCD image). It can however, be measured directly by applying simple trigonometry. The diagram below shows the apparent shift of a nearby star to an observer on the Earth as it orbits the Sun.
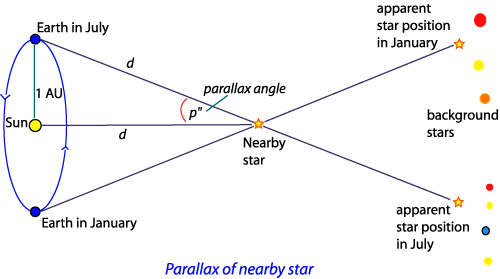
The diagram above shows how a nearby star appears to shift relative to more distant background stars.
CAREFUL: This diagram IS NOT TO SCALE. In reality the triangle formed by the Earth at July, the Sun and the nearby star is much narrower as even the nearest star is at a large distance. The parallax angle, p, is extremely small. For triangles with one very small angle we can apply the small angle approximation:
tan p ≈ p.
This then means that the Earth - star and Sun - star distances are the same, that is d.
As the parallax angle for even the nearest stars is very small we use the unit arcsecond (also written as arcsec, as or "). There are 60 arcsecs in 1 arcminute and 60 arc minutes in 1 degree of arc.
∴ 1 arcsec = 1/(60x60) = 1/3600th of a degree.
By way of comparison, the Moon subtends an angle of about ½° or 30 arcminutes. Therefore the Moon has an angular diameter of 30 x 60 = 1800 arcsec.
So how do we actually use parallax (strictly speaking we call this trigonometric parallax) to find the distance to stars?
The Parsec
The closer a star is to us, the larger its angle of parallax will be. Astronomers have defined a standard unit of distance to be the parsec (pc). One parsec is the distance to a point in space that subtends a parallax angle of one arc second. This produces the simple but effective relationship:
or
d = 1/p
By measuring the parallax angle for a star, astronomers can then directly determine its distance. In reality it is not quite so simple for several reasons. The angle of parallax for even the closest stars is always < 1.0 arcsec so careful observation and precision is required. Corrections have to be made for atmospheric refraction and the effects of "seeing". Also stars actually do appear to move across the sky relative to other stars in a definite direction over time. This is called proper motion and must be accounted for when determining parallaxes. If you study the diagram above you will see that the greatest baseline ground-based astronomers can obtain is by observing the star at times six months apart. This gives a baseline of two astronomical units and an angle of 2p.
So how far is a parsec?
Many people think that astronomers measure distances in light years. Recall that a light year is simply the distance that light travels in one year. As light travels at 300,000 km per second in a vacuum, the total distance light travels in a year is approximately:
3.0 × 10^8 × 60 × 60 × 24 × 365 ≈ 9.46 x 10^15 m or almost 10 million million km.
1 parsec ≈ 3.26 light years
Why do astronomers use the parsec and not the light year? Well the parsec is directly determined from geometry and trigonometry and does not depend upon an arbitrary time scale. If it is possible to measure an object's parallax, its distance can be directly and quickly calculated using expression 2.1 above.
How far are the stars?
Apart from the Sun, currently the closest star to us is a small red dwarf M5 class star, Proxima Centauri. It has a parallax of 0.772". Therefore its distance is:
so d = 1/0.772
∴ d = 1.30 pc
This corresponds to a distance of 4.24 light years. All other stars are even further away. Sirius, the brightest star in our night sky has a parallax of 0.375" ∴ is 2.7 pc distant. Our Sun is about 8 kpc, ie 8,000 parsecs from the centre of our galaxy, the Milky Way. Its nearest large neighbour, the spiral galaxy M 31 or the Andromeda Galaxy is 725 kpc.

