Using Binary Stars to Determine Stellar Masses
Binary stars are of great importance to astronomers because they provide virtually the only means of directly determining the masses of stars other than our Sun. As mass is such a key property of stars and to a large extent knowing a star's mass determines its life cycle and fate, being able to accurately determine stellar masses is vital in refining our models of stars.
Kepler's Laws
To find the mass of a binary system we need to apply Kepler's Laws. If we adapt them for a binary system where the masses of the component stars are similar then:
- The stars orbit each other in elliptical orbits, with the centre of mass (or barycenter) as one common focus.
- The line between the stars (the radius vector) sweeps out equal areas in equal periods of time (sometimes called the Law of Equal Areas).
- The square of a star's period, T, is directly proportional to the cube of its average distance from the centre of system mass, r:
T2 ∝ r3. This is the Law of Periods or Harmonic Law.
Let us now see how we can apply these to determine the total system mass and the mass of the individual component stars. We shall refer to the diagram below.
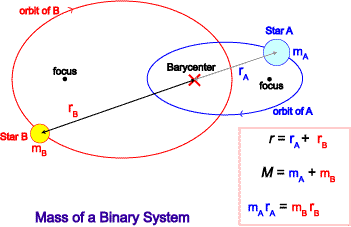
Deriving Equations for Mass of Binary System
The barycenter or centre of mass of the system is where:
and as r =rA + rB (5.2)
then rB = r - rA
so mArA = mB(r - rA)
∴ rA = mBr/(mA + mB)
or rA = mBr/M (5.3) where M is the total system mass.
The forces acting on each star are balanced, that is the gravitational force equals the centripetal force so;
GmAmB/r2 = mAv2/rA (5.4)
where v is the orbital speed of A.
Unless v can be measured or inferred directly from Doppler shift in its spectrum it must be calculated from the period, T:
v = 2πrA/T
so substituting this into (5.4) gives:
GmB/r2 = 4π2rA/T2
so if we then substitute in (5.3) we get:
GmB/r2 = 4π2mBr/T2M or:
M = 4π2r3/GT2 (5.5)
which can be rewritten as:
mA + mB = 4π2r3/GT2 (5.6)
(This is the form specified in the HSC formula sheet)
now (5.5) is simply an expression of Kepler's 3rd Law;
r3/T2 = GM/4π2 (5.7)
Using equation 5.5 or 5.6 we can determine the mass of the binary system if we can measure the orbital period and the radius vector (separation between the two components) for the system. In practice most systems will not have their orbital plane perpendicular to us so we need to adjust for the observed inclination.
Whilst it is relatively straight forward to determine the total system mass, it is harder to determine the individual masses of the component stars. This requires the distance from a component star to the barycenter to also be measured. We can then use this to determine the mass of that star by using:
Once the mass of one component and the total system is known it is straightforward to calculate the mass of the other component.
Examples of Mass Calculation in Binaries
Example 1: Determining the total mass of a system.
The α Centauri system is 1.338 pc distant with a period of 79.92 years. The A and B components have a mean separation of 23.7 AU (although the orbits are highly elliptical). What is the total mass of the system?
To solve this we use equation 5.5 but before we simply substitute in we need to check units. Whilst we can use use AUs and years to give us a relative value when applying Kepler's Laws, if we want a full numerical solution we must convert all parameters to S.I. units as the constant G is normally expressed in such units (G = 6.672 × 10-11m3.kg-1.s-2).
r = 23.7 AU = 23.7 × 1.50 × 1011 m = 3.55 × 1012 m.
Now substituting these into (5.5);
M = 4π2r3/GT2 gives:
M = 4π2(3.55 × 1012)3/((6.672 × 10-11) ×(2.522 × 109) 2)
∴ M = 4.162 × 1030 kg.
Example 2: Calculating the mass of one of the component stars.
As α Cen is a nearby visual binary system, careful astrometric observations reveal that the primary component, α Cen A has a mean distance of 11.2 AU from the system's barycenter. What is the mass of each of the component stars in the system?
Now distance rA = 11.2 AU = 11.2 × 1.50 × 1011 = 1.680 × 1012 m.
We shall use this to first find the mass of α Cen A.
Using equation (5.8):
mA = M(r - rA)/r
and substituting in:
mA = 4.162 × 1030(3.55 × 1012 - 1.680 × 1012)/3.55 × 1012
∴ mA = 2.192 × 1030 kg.
so α Cen A has a mass of 2.192 × 1030 kg.
Now to find mass of α Cen B we simply use M = mA + mB so that:
mB = M - mA
mB = 4.162 × 1030 - 2.192 × 1030
∴ mB = 1.970 × 1030 kg.
so α Cen B has a mass of 1.970 × 1030 kg.
The mass of α Cen A makes it about 1.1 × that of our Sun. It, too is a G2 V star with a luminosity about 55% greater than that of our Sun. α Cen B is a dimmer K0-1 V min sequence dwarf with 90% of the Sun's mass and only about half as luminous.
Of particular value to astronomers are systems that are both eclipsing and spectroscopic. The radial velocity values from the spectral data can be used to calculate absolute rather than just relative values for the stellar radii. This can then be combined with orbital inclination parameters obtained from the light curve to give the stellar masses and mean stellar densities. The relative luminosities and total luminosity of the system can be derived then used to calculate the total flux of the system. This then allows us to calculate the distance to the system. We can also infer the mass and luminosity of each star.
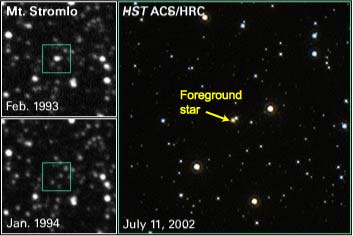
As already mentioned, binary stars are of vital importance as they allow us to determine stellar masses. To date (August 2004) only one single star other than our Sun has had its mass accurately determined by a means unrelated to Kepler's laws. Astronomers involved in the MACHO project at Mt Stromlo took an image in 1993 that showed microlensing of a distant background star by a closer star in the foreground. Recent parallax measurements and observations by the HST have allowed astronomers to calculate that the faint, red foreground star has a mass only one-tenth that of our Sun. For more information, read the press release.