The Colour of Stars
Introduction
When we gaze up into a clear, dark night sky we can see a few thousand of the many billions of stars in our Milky Way galaxy. Most appear as dim points of white light. A few of the brighter ones show hints of colour such as orange or blue. All these stars are incredibly distant from us. Direct measurements using trigonometric parallax show that α Centauri, the closest star system to us other than our Sun, is about 4.3 light years away. This is far too distant for us to visit it by spacecraft so how is it possible to study stars in detail?
Most of the information we gain about stars comes through the study of the electromagnetic radiation they emit. Astronomers gather the starlight in telescopes then use a variety of scientific instruments to analyse the light. Since the end of the Second World War astronomers have been able to study wavebands other than visible light. Some wavebands, such as radio and visible light can take place from the ground but others such as X-ray and ultraviolet require telescopes in space to overcome atmospheric absorption.
Have a look at a rich starfield in our galaxy:

A starfield in the region of Sagittarius showing stars of different colours.
These stars seem to vary in colour, some appear white, others orange while a few are bluish. This tells us something about the nature of the individual stars. The stars also seem to vary in size. In fact all these stars are too distant to be seen as actual disks so the variation in size in this image is purely an indication of the relative brightness of the stars; the brighter they appear, the larger the image.
Colour and Temperature
Study the photos below. Which one is hotter, A or B?


We tend to associate the colour red with heat or warmth and blue with cool or cold partly because red indicates fire and blue, ice. This could confuse us when we study stars. Which colour of star, red or blue, is actually hotter? The answer to this question can be found in the photos of flames from a bunsen burner at right.
From junior science classes in high school we learn that a blue bunsen flame is hotter than the orange "safety" flame. This relationship holds true for stars as well. Stars are massive spheres of gas, mostly hydrogen and some helium. They produce energy through thermonuclear fusion in their cores. Despite their complexity and size we can analyse some of their properties by adopting a simpler model. One such useful concept is that of a blackbody.
Blackbodies
A blackbody is a theoretical object that absorbs all the electromagnetic radiation and energy that falls onto it. Because it reflects no light it would appear black (hence blackbody) if cold but as it absorbs energy it heats up and radiates it away into the surrounding space. The hotter it is, the more energy it emits.
Using the example of a metal rod held into a hot flame consider what would would happen if you removed it after a very short time. You may feel that the rod has heated up due to the infrared radiation emitted from the rod and felt by your hand when it is near it but the rod does not appear to glow. If you held the rod in the flame for longer before removing it it may start glowing a dull red colour. It now emits some visible light in addition to the infrared. Holding it in the flame for even longer may see it glow orange then yellow-hot. It would also feel much hotter when removed. If you held it in a hot enough flame for long enough it may even glow white-hot or blue-hot. The colour of a blackbody radiator therefore tells us something about its temperature. An object that is blue-hot actually has a higher temperature than the same object if it was red-hot.
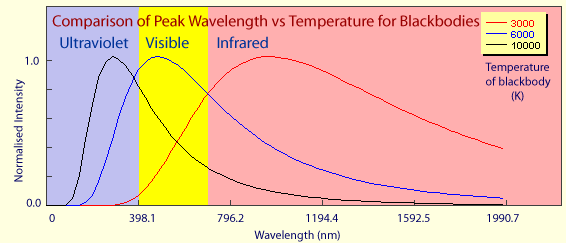
By measuring the intensity of energy emitted at each wavelength from a blackbody we can produce plots such as those shown below. In this diagram we can compare the emission from a blackbody at four different temperatures. What do you notice about the shape and size of each plot?

The above curves are called Planck blackbody curves after the scientist, Max Planck who developed a theoretical explanation for them in 1900. If you compare the plots for different temperatures several points are apparent.
- The hotter the blackbody is, the shorter the wavelength at which the emitted radiation peaks. In the above example, the 6,000 K object has peak emission at around 500 nm, well in the visible band whereas the 4,000 K object peaks at just below 780 nm in the infrared.
In the plot below the energy intensity has been normalised to a maximum of one for each object. This allows us to compare the peak wavelength from objects of widely differing temperatures more clearly. The 10,000 K blackbody actually has peak emission in the ultraviolet part of the electromagnetic spectrum, the6,000 K one in the visible and the 3,000 K object peaks in the infrared.
 Credit: Adapted from an applet by M. Guidry.
Credit: Adapted from an applet by M. Guidry. - The hotter the object is, the more energy it emits at every wavelength than the same object at a cooler temperature. The 6,000 K object emits more energy at 800 nm than the 4,000 K one even though the cooler object peaks near this wavelength.
- From 2. above it follows that the hotter object emits more energy in total than a cooler one. The total energy emitted can be expressed as a function of the area under the curve. The area beneath the 6,000 K curve is greater than that under the 4,000 K curve.
Effective Temperature of a Star
How does all this help us study stars? If we are able to measure the intensity of emitted radiation from a star at different wavelengths we can try to plot and fit a Planck curve for it. Strictly speaking this is only valid if stars are blackbodies. In fact a star approximates a blackbody quite well even if its actual spectral curve is not a perfect fit. Once we have a spectral curve for the star we can determine the wavelength at which emission peaks. Wien's law is name of the direct relationship between peak wavelength and temperature. Applying this allows us determine the equivalent blackbody temperature for the star. This is called the effective temperature of the star and is a measure of the surface temperature. The diagram below shows a spectral curve for a star and how Wien's law can be used to determine the effective temperature. Note how the spectrum for the star approximates the shape of a Planck curve from a true blackbody. Most stellar spectra also exhibit absorption lines, shown here as dips in the spectrum.
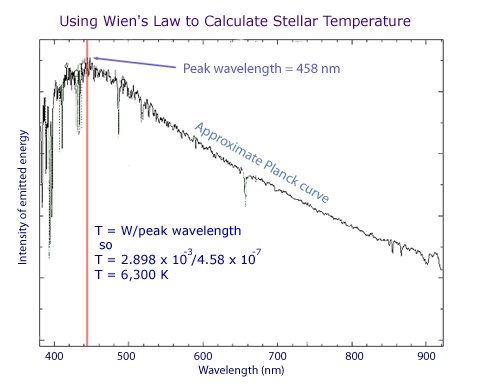
λmax = W/T
where W is a constant = 2.898 × 10-3 for T in kelvins and λmax in nm.
(*Not required in syllabus for The Cosmic Engine)
Our Sun has an effective temperature of 5,770 K and a peak emission wavelength of 550 nm. Even without a full spectral curve for a star astronomers can determine its effective temperature by comparing its brightness through two or more filters that only allow certain wavebands through. This technique allows the temperatures of many stars in a field to be determined quickly using only a few exposures in contrast to the need to obtain separate spectra for each star for the method using Wien's law.
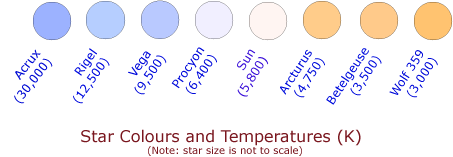
Star Colours
The colour of a star therefore is a function of its effective or surface temperature. Effective temperatures range from a few thousand degrees up to 30-40,000 K for a few very hot, blue stars. The diagram below shows the colour of some well known stars with a range of effective temperatures. The colours are correct for rgb monitors.